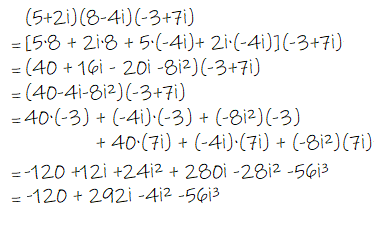Imaginary and Complex Numbers

When you first learn about square roots, the teacher lays down the law: never take the square root of a negative! However, a few years later in your math career, they reveal that, as with Santa Clause and the Tooth Fairy, the truth has been hidden from you all along. You actually can take square roots of negative numbers - they're called imaginary numbers.

So what the heck are imaginary numbers anyway?

Imaginary numbers are numbers that are multiples of the imaginary unit $i$, which is defined as $\sqrt{-1}$. If you've got $i$ times a real number (a.k.a. any of the "normal" numbers that you've learned up to this point), then you've got an imaginary number. For example, $5i$, $-2i$, $\frac{7}{4}i$, $8.568394i$, $\sqrt{15}i,$, and $\pi i$ are all imaginary numbers.

A complex number is any number that's a combination of a real number plus an imaginary one. For example, $12 - 3i$ is a complex number because it's a real number ($12$) plus an imaginary number ($-3i$).

Also, every complex number has a conjugate, another complex number that you can multiply it by to get a real number. (Think of it like the number's soul mate - it makes it complete!) The conjugate of any complex number has the same real part, and the opposite imaginary part. For example, the conjugate of $7 - 2i$ is $7 + 2i$: the real part ($7$) is the same, while the imaginary part ($-2i$) switched from negative to positive. Similarly, the conjugate of $-15 + 6i$ would be $-15 - 6i$: the real part $-15$ didn't change, but the imaginary part switched from positive to negative.

Ok, so that's what they are, now tell me how to use imaginary numbers.

One way that you'll use imaginary numbers is in situations where you have to simplify the square root of a negative expression. For those problems, you can factor out a negative one, then simplify $\sqrt{-1}$ and the rest of the square root separately from each other.

For example, consider $\sqrt{-192x^5y^7}$. First, we can break that up into $\sqrt{-1} \cdot \sqrt{192x^5y^7}$. Then, $\sqrt{-1}$ becomes $i$, so we have $i \cdot \sqrt{192x^5y^7}$. Then we can simplify the other square root like normal, giving us a final result of $8x^2y^3 \sqrt{3xy} i$.

When you're doing problems that involve imaginary and complex numbers, they'll typically just use the same principles that you've used when solving problems with real numbers and variables in algebra, with a couple exceptions. Most of the time, you can work with $i$ the same way you would with $x$ or $y$ or any other variable; you just have to make sure you're accounting for the fact that $i$ really means $\sqrt{-1}$.

The biggest way that this can come into play is in problems that end up with exponents of $i$: $i^2 $ is really $\sqrt{-1}^2$, which is just -1, so anytime you have $i$ raised to an exponent (such as $i^2$ or $i^7$), you'll need to simplify it to get rid of the exponents.

For example, suppose you needed to simplify $(5+2i)(8-4i)(-3+7i)$. The first step would be to multiply it out using the distributive property, just as we would if $i$ were $x$ or any other variable. This results in $-120 + 292i -4i^2 -56i^3$.

Next, we apply the $i^2 = -1$ rule. That means $-4i^2$ becomes $-4(-1)$, or $+4$. On the next term, $-56i^3$ is the same as $-56i·(i^2)$, so it becomes $-56i(-1)$, or $+56i$. So now we have $-120 + 292i + 4 +56i$. Then, we combine like terms, giving a final result of $-116 + 348i$.

The other way that simplifying problems with complex numbers can get tricky is in cases where you have a complex number on the bottom of a fraction. When that happens, you have simplify the fraction so that you have $i$ in only the numerator, so the real and imaginary parts can be split into separate terms. We do this by multiplying the top and bottom of the fraction by the conjugate of the denominator.

For example, consider the problem $\frac{3+2i}{4-7i} + 6i - \frac{15}{i}$. Since the two fractions each have an $i$ in the denominator, we need to move the complex numbers to the numerator, by multiplying by the conjugate.

For $\frac{3+2i}{4-7i}$, we need to multiply by the conjugate of $4-7i$, which is $4+7i$. This gives us $\frac{(3+2i)(4+7i)}{(4-7i)(4+7i)}$. When we multiply that out, we end up with $\frac{-2+29i}{65}$.Similarly, on $\frac{15}{i}$, we multiply the top and bottom by the conjugate of $i$, which is $-i$, and multiply it out, resulting in $-15i$. Next, we split $\frac{-2+29i}{65}$ into its real and imaginary parts: $\frac{-2}{65}$, and $\frac{29}{65}i$. Finally, we can combine like terms, giving us $\frac{-2}{65} + \frac{1394}{65}i$.

Now you try! Test your knowledge with the following problems, and let me know your answer in the comments:

Evaluate $\sqrt{-72x^5}$.

Simplify $(5-7i)(-3+2i)$.

Simplify $\frac{8+4i}{6-9i}$